Achievement
Successfully simulated scalar quantum field theory model on IBM QPU. Part of this work used XACC
Significance and Impact
This work applies quantum computing to quantum field theory and leveraged the XACC programming framework.
Research Details
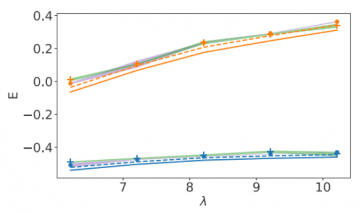
- Computed ground state energy and excited state to infer characteristic mass gap
- Implemented error mitigation protocols to enhance our results.
Overview
Quantum field theory (QFT) simulations are a potentially important application for noisy intermediate scale quantum (NISQ) computers. The ability of a quantum computer to emulate a QFT therefore constitutes a natural application-centric benchmark. Foundational quantum algorithms to simulate QFT processes rely on fault-tolerant computational resources, but to be useful on NISQ machines, error-resilient algorithms are required. Here we outline and implement a hybrid algorithm to calculate the lowest energy levels of the paradigmatic 1+1–dimensional ϕ4 interacting scalar QFT. We calculate energy splittings and compare results with experimental values obtained on currently available quantum hardware. We show that the accuracy of mass-renormalization calculations represents a useful metric with which near-term hardware may be benchmarked. We also discuss the prospects of scaling the algorithm to full simulation of interacting QFTs on future hardware.
Last Updated: January 15, 2021 - 1:55 pm